by Jan Boyd
As artists, we often come across references to the related concepts of Fibonacci numbers and the Golden Mean. What do these terms mean, and are they useful in creating botanical artwork?
These concepts are quantifiable, identifiable and fascinating. They can be identified in and related to the beauty we find in flowers, trees, fruit and vegetables, in paintings, in sculptures, in music, in poetry, in the human body and more. For this short article I will concentrate on how they can impact our botanical artwork in a meaningful way.
For a more thorough presentation you can view my Youtube video.
Beauty is said to be in the eye of the beholder, but in studying the beauty of nature, art and architecture, we can discern a common principle – the universal experience of pleasant proportion. This basic understanding of proportion has been recognized since antiquity and named by philosophers and mathematicians variously as the Golden Proportion, Golden Ratio, Golden Section or Golden Mean. All these terms (which can be used interchangeably) represent what can be reduced to a mathematical equation, which identifies and quantifies proportions that please the eye. In everyday life we use the word “proportion” to indicate a comparative relationship between parts of entities with respect to size or quantity; parts of a picture, of an object or person, or of a building.
As seen in the pictures below, the use of the Golden Mean within a painting creates pleasing, proportional relationships. These relationships are characterized by pairings of Fibonacci numbers. Each relationship seen in the chart is a pair of Fibonacci numbers whose relationship to one another is approximately 1:1.618 (8 divided by 5 = 1.6, 5 divided by 3 = 1.666…). A Golden rectangle has sides that are in the Golden Ratio.
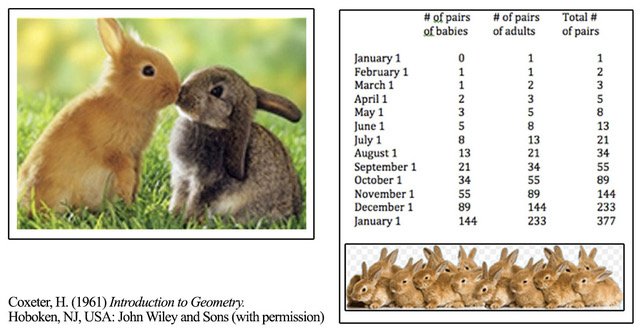
So where does Fibonacci come into this? At the dawn of the 13th century, the Middle Ages were fading and glimmers of the Renaissance were starting to appear. Leonardo Pisano, a brilliant Italian mathematician (later to be known familiarly as Fibonacci) wrote his most well-known work, Liber Abaci (Book of Calculating), in which he forever changed Western methods of calculation. In this treatise he raised the following hypothetical question that has become known as the first algebra word problem.
What would happen if a pair of rabbits were put into an enclosure to breed? How many pairs of rabbits will there be after a year if it is assumed that every month each pair produces one new pair, which begins to bear young two months after its own birth? It was not the answer 377 that was most interesting, but the emergence of the pattern by which this could be solved: 0, 1, 2, 3, 5, 8, 13 …… the string of numbers that begins with 0, 1 where each subsequent number is the sum of the previous two numbers. This is credited as the first known written record of what later became known as the Fibonacci sequence.
Over the centuries, mathematicians, botanists, artists and philosophers have noted the existence of this sequence in diverse disciplines from pine cones to poems, sunflowers to symphonies, ancient art to modern computers, the solar system to the stock market!
One of the most fascinating examples of the sequence in the animal kingdom is the spiral that characterizes much animal growth. The spiral below demonstrates the construction of the logarithmic spiral – a long slow spiral characterized by all radii from its center intersecting the spiral at exactly the same angle. Using the Fibonacci sequence, the spiral grows to accommodate the growing animal inside in a nautilus shell – and additional examples can be seen in the human ear and in the human fist.
Another interesting note in animal growth and form is the presence of the Fibonacci number 5 and the Pentagon shape, which has Fibonacci elements, as can be noted in the diagrams below. The shape is visible in the radial symmetry of some plant and animal growth from starfish to sand dollars; from the 5-star pattern of carpels visible inside a transversely cut apple to sea urchins and 5-petaled flowers.
So how does all of this relate to the work we, as botanical artists, observe and illustrate? In external plant structure, botanists have found that Fibonacci numbers show up often in plants that have multiple parts (leaves, sepals, petals, etc.) arranged around a single stem. If you count the number of petals in the predominance of flowers, you will find that the total is a Fibonacci number. For example, an Iris has 3 petals, flowering quince has 5, and delphinium or cosmos have 8. A ragwort has 13 petals; an aster 21 and Michelmas daisies have 55 or 89.
Internal growth patterns in plants also exhibit Fibonacci numbers. By examining the center of a sunflower, a pattern of two spirals is evident. In a typical sunflower there will be 21 or 34 running clockwise and 34 or 55 running counterclockwise – all Fibonacci numbers. The florets are packed into the tightest possible area that will allow each to receive optimal nutrients and sunlight. Pinecone growth shows that they typically have 5 or 8 clockwise and 8 or 13 counterclockwise spirals in the bracts that grow out of the stem.
Plant growth patterns are another area where Fibonacci patterns can be observed. Plants grow toward sunlight, growing in spirals as they reach for moisture, sunlight and air, which are found to be available cyclically. The tip of a branch moves in a somewhat circular motion as it lengthens and grows. As a branch grows it generates leaves in fractions of a full turn of the branch (after 2/5 or 3/8 or 8/13th of a full cycle for example) rather than at equal intervals, so that new leaves are not directly over earlier growth, which would otherwise tend to deny them of the nutrients needed to continue to grow. These phyllotactic ratios (Phyllotaxis) are usually Fibonacci numbers. In the diagram below eight stems were produced in three complete growth spirals.
We now understand these principals in regard to plant growth and structure but there is one more very important aspect to consider as we create our illustrations – the appreciation of Fibonacci numbers and the Golden Mean as they relate to artistic layout.
Over the years I have had the opportunity to study with one of the world’s most renowned calligraphers, Sheila Waters. She teaches the following for layout in lettering which we can apply to our work with flowers, plants, etc. as well:
All of this information can inform our understanding of the plants we paint – both the plants themselves and the understanding of beautiful proportions in the layout on our paintings. Using the Golden Mean and associated Fibonacci numbers, thereby avoiding even proportions both vertically and horizontally, will help to keep paintings from appearing static and dull.
Fibonacci numbers can inform the numbers of foci in a painting – quantities of 1, 3 or 5 are generally perceived as more pleasing to the eye. Awareness of balance issues can help to avoid images that might appear out of proportion.
So now it might be helpful to see these principals in practice by applying them to an Orchid painting I created a few years ago. I believe that it is in reasonable Golden Section proportions. In addition to the general layout, it is also interesting to note that there are 5 large leaves, 3 smaller leaves and 3 flowers at the top. Also note that each orchid has 5 tepals and one lip…. All Fibonacci numbers!
While the value of knowing these concepts may now be manifest, it is also wise that they not be followed slavishly in our field of fine art. “Perfect” faces, if we all had them, would look robotic and unnatural. Flowers and plants certainly have strong tendencies to conform to these concepts, but their variations are what make them interesting and always fascinating. The best use of Fibonacci numbers and the Golden Mean in botanical illustration, in my opinion, would be to be mindful of them when creating a piece of art and very alert to how they might help your analysis if you create or view a piece of art that seems “off” somehow.